회사소개
찾아오시는 길
연혁
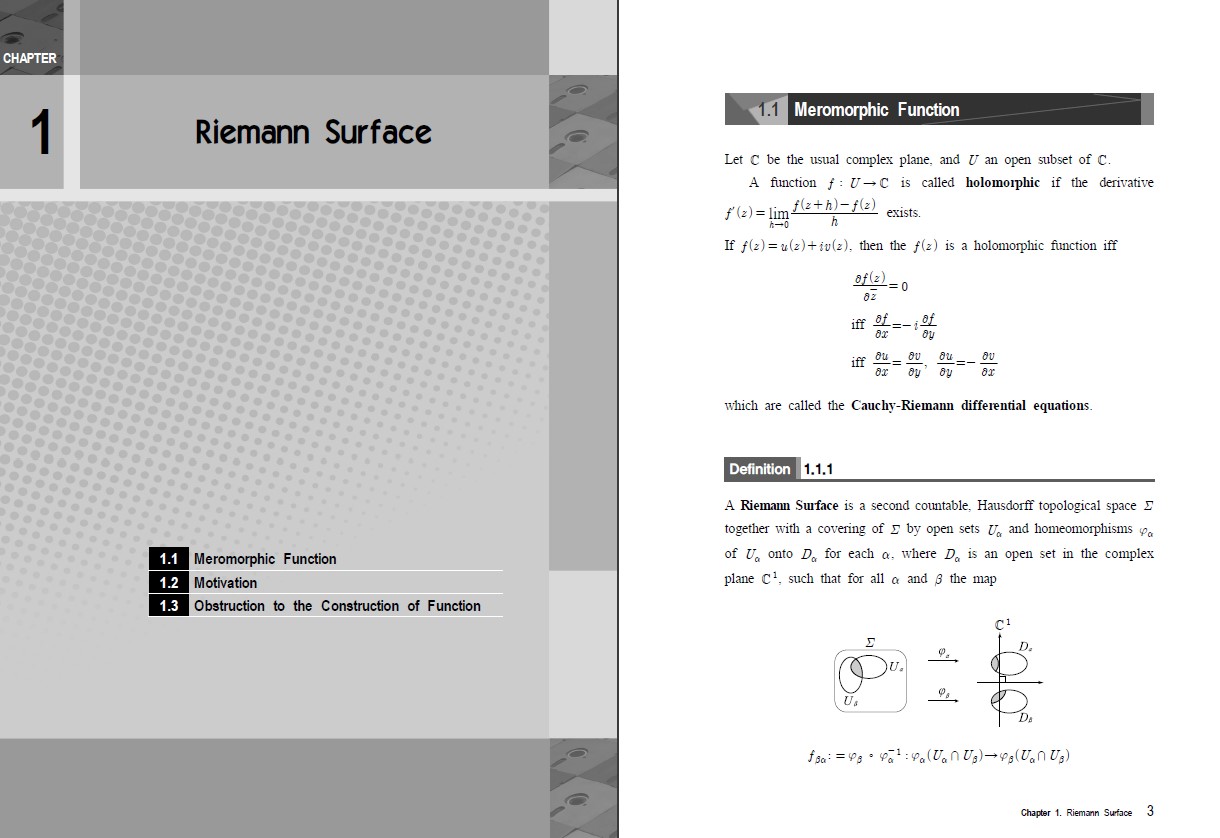
수학
수학교육학
통계학
공학
언어/영어
경영경제
단행본
ebook
수학
수학교육학
통계학
공학
언어/영어
경영경제
단행본
재정가 도서
저서 문의
번역서 문의
단체구매 문의
오탈자 문의
기타 문의
마이페이지
장바구니
위시리스트
도서후기
도서문의